A strange 9-limit temperament
Dave Keenan, 15-Nov-1998
last updated 23-Feb-2000

I put this chart at the top to get you interested. It's explained later.

Paul Erlich's 7-limit challenge* has led me into some strange regions of tuning space, but none stranger than this one. This is my best and final attempt to find a tuning with 12 notes per octave or less, that approaches the proportion of 7-limit harmonies of his 10 of 22-tET (or twin chains of similar sized fifths). Unfortunately it fails his melodic criteria for being a generalised diatonic, but in numbers of usable harmonies I am almost certain that the tuning I am about to present is the best possible 12-tone 7-limit tuning which is distinct from 12 of 22-tET, and the best possible 12-tone 9-limit tuning. Of course this depends on your idea of usable harmonies, i.e. acceptable errors.

*See Paul Erlich's article Tuning, Tonality, and Twenty-Two-Tone Equal Temperament.

While its 12 tones only give 8 complete 7-limit tetrads, no better than any 12 tone tuning with twin chains of meantone-sized fifths, it has 4 additional 7-limit triads (5:6:7 and 1/7:1/6:1/5) and 4 additional 5-limit triads. What's more, 4 of the 8 tetrads and all of the 7-limit triads can be extended to 9-limit pentads and tetrads respectively.

So from a 9-limit perspective what we really have is 4 complete 9-limit pentads, 4 complete 7-limit tetrads, 4 9-limit tetrads (5:6:7:9 and 1/9:1/7:1/6:1/5) and 4 5-limit triads. There are equal numbers of otonal (major) and utonal (minor) in all cases.

Many thanks to Graham Breed, who finally got me to see this tuning as a "detempered" 12 of 22-tET. That is, taking 12 of 22-tET part of the way back towards Just. Not that it was in any way obvious how to do this profitably. In fact I arrived at this tuning by starting with meantone sized fifths.

Detempering would normally mean increasing the accuracy of intervals (ratios) at the expense of a reduction in the number of sufficiently accurate intervals. But in this case, while we do lose four 7-limit intervals, we might be considered to make up for it by gaining some 9-limit ones.

This tuning looks like two chains of fifths a half-octave apart, where the middle fifth of each chain is slightly wider than a 22-tET fifth and the others are slightly narrower.

As offsets from 12-tET it is:

Consider the harmonic resources in each case.

12 of 22-tET has 12 complete 7-limit tetrads.

In 12-tone 7-limit JI, the most we can get appears to be 6 complete tetrads plus 2 triads (but with no pretense of being a melodic construct). Thanks to Carl Lumma for that.

In the 7-limit, this temperament is intermediate, with 8 complete 7-limit tetrads and 4 others that have a broken 4:7 (and so become 8 triads). However these bad 4:7's are actually good 9:16's which occur as 4:9's in extending other tetrads to 9-limit pentads. So no harmonic resources are wasted relative to 12 of 22-tET. In fact when 9-limit is considered, this temperament has more usable intervals than 12 of 22-tET. Notably, 5:9's essentially do not exist in 22-tET. However a fairer comparison is with twin chains of uniform fifths which are optimised for 9-limit ratios. This tuning has fifths of 708.13 cents [(2¹¹ ´ 3⁴ ´ 5^-2 ´ 7^-2)^1/12].

Here's a comparison of the errors in cents for the various intervals:

Note that only in the 6:7's, and in 2 of the 10 fifths, is there a significant increase in error. The minor thirds, the other 8 fifths, and the 9-limit intervals except the 7:9, are significantly improved. Although the 7:9 gets worse, it is still only a 5.1 cent error. So we've gained some accuracy with little loss of 7-limit or 9-limit harmonic resources.

The table refers to the version of Strange9 tuning having 706 and 712 cent fifths. Whether you use maximum error magnitude (max absolute error) or RMS error as your overall error measure, there is an error minimum in the vicinity of this pair of fifth sizes.

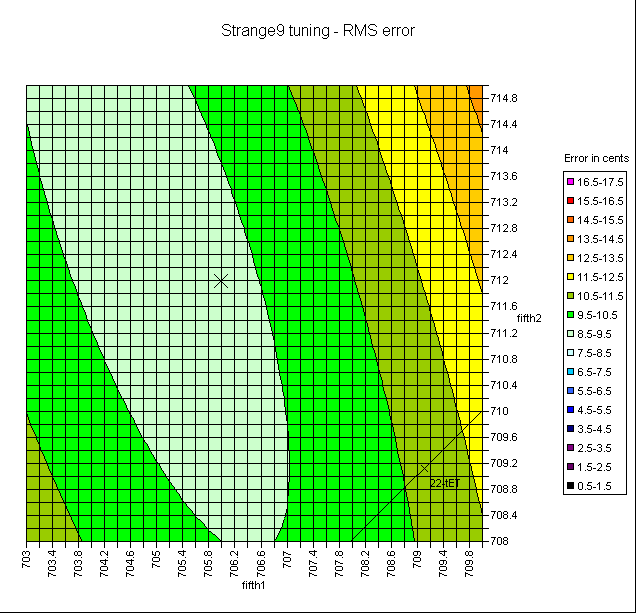
See the chart above, which shows, for a given pair of fifth sizes, the maximum error over all 9-limit intervals excluding the 5:7 (which is always 17.5 cents). The small islands and the zig-zag purple edge are artifacts of Excel's interpolation algorithm. The long line shows a gently sloping valley. The X shows the favoured version, which was moved up this valley just enough to take the second fifth out of the 'wolf' category. The short line is where the two fifth-sizes are equal. The position of 22-tET is shown on this line. Where the two lines meet is the optimum fifth-size (708.13 cents) when there is only one size of fifth. This differs slightly from Paul Erlich's equally-weighted optimum only because (a) he uses RMS (or sum of squares) instead of maximum error, and (b) he is considering 7-limit, not 9-limit.

To see the 7-limit case or the error surfaces for the individual intervals or to experiment with different error weightings, download this Excel 97 spreadsheet (81 kB) which was used to generate the above chart.

This temperament can be seen as simultaneously approximating the following Just tunings.

Then there's the question of modulation. Paul Erlich's 7-limit decatonic scales would presumably occur in only one or two positions in a suitable 7-limit 12-tone JI. They occur in 4 positions in 12 of 22-tET. They sort of occur in 4 positions in this temperament, however two of these have the transmuted 4:7's in the otonalities while the other two have them in the utonalities.

Here's some more about Strange9 tuning, including chord charts.